Integrate Area Under Curve

This gives you the area between the curve fof x23x4 and x-axis between x1 and x3. Few of the basic steps for using this calculator are.

How To Understand Calculus Integration Rules And Examples Calculus Studying Math Ap Calculus

3 1s23x4dx x2 3 3x2 2 4x2.

. This will add a rectangle onto the plot. Ribet IntegrationArea under a curve. The curve is completely ABOVE the x -axis.

When Δ x becomes extremely small the sum of the areas of the rectangles gets closer and closer to the area under the curve. 822 Integrating Other Functions Trig ln e etc 823 Reverse Chain Rule. Select whether you want to evaluate the area under the curve functions as per definite integral or indefinite integral.

If latexvtlatex represents the velocity of an object as a function of time then the area under the curve tells us how far the object is from its original position. The actual function of the integration is to add up all of these individual rectangles we talked about above so that we can find the total area underneath the curve fx ie. This method will split the area between the curve and x axis to multiple trapezoids calculate the area of every trapezoid individually and then sum up these areas.

Sum all area values to find the total area under the. In mathematics an integral assigns numbers to functions in a way that describes displacement area volume and other concepts that arise by combining infinitesimal data. Thus compute the area using definite integrals by subtracting them from one and another.

We know that cos0 sin so cost has derivative sint. Hence Z ˇ 0 sinx dx sint cos ˇcos0 2. 814 Area Under a Curve.

For this we need to find a function whose derivative is sin. In this section we start off with the motivation for definite integrals and give one of the interpretations of definite integrals. Area under curve x 2 3 x 4 between x1 and x 3 3 1 s 2 3 x 4 d x 28667 sq.

Go to the Baseline tab. Enter the area formula starting from the second row. The formula will refer the data points in the same k and the.

815 Area between a curve and a line. Between the curve and the x-axis. Integrate from the Origin menu when the graph window is active to bring up the Integrate.

So we integrate it between x1 and x3. Click the OK button. 14 a For the function.

Calculate the area under y sinx from x 0 to x ˇ. Trapezoidal Rule is a rule that evaluates the area under the curves by dividing the. Then you can drag the AutoFill handle of the formula cell.

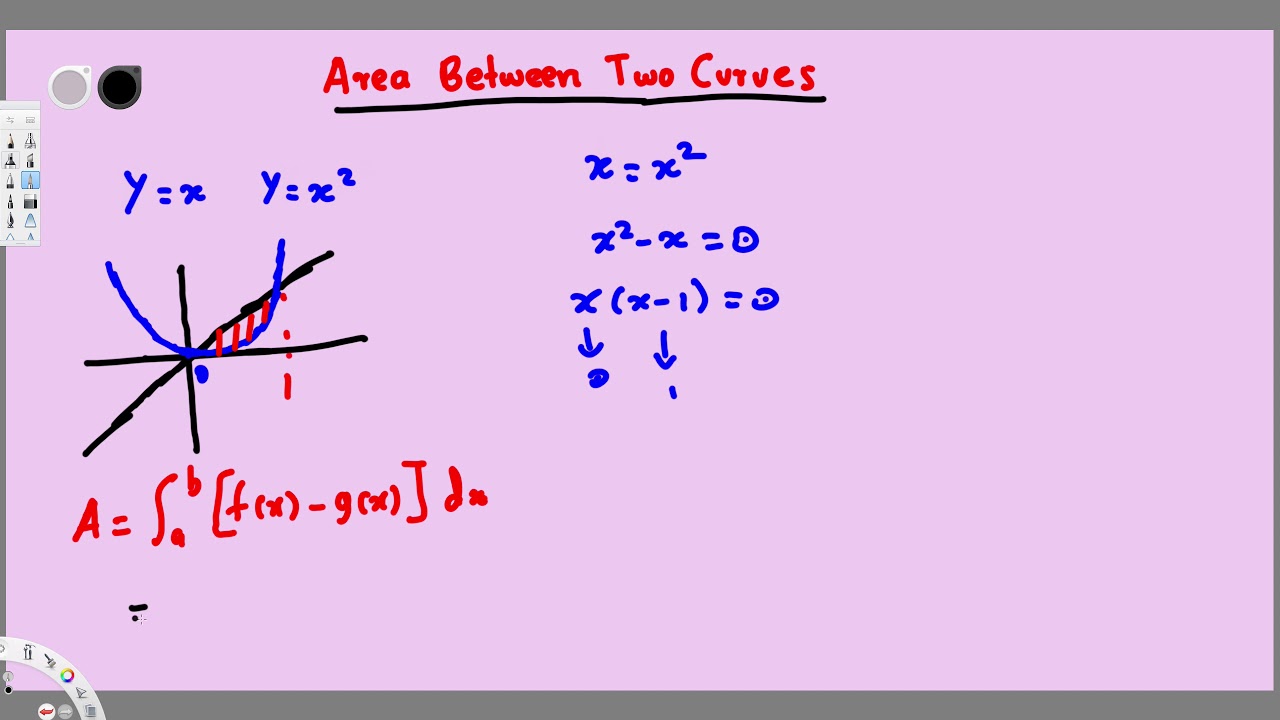
The process of finding integrals is called integrationAlong with differentiation integration is a fundamental essential operation of calculus and serves as a tool to solve problems in mathematics and. Choose Constant Y for the Method and then enter 2 in the Y edit box. Hence we can say that Area under the curveabUpper Function Lower Function dx.

Calculate the area under y sinx from x 0 to x ˇ. Thus the area can be computed using the above formula. A Level Maths revision tutorial videoFor the full list of videos and more revision resources visit wwwmathsgeniecouk.

The first trapezoid is between x1 and x2 under the curve as below screenshot shown. Find the area of. The integration area is shown at the top of the rectangle.

The first step is to enter the function from the calculator or load the example. If it actually goes to 0 we get the exact area. What is the equation for the area under the curve from y.

This calculator will help in finding the definite integrals as well as indefinite integrals and gives the answer in a series of steps. F x x23x4. Ribet IntegrationArea under a curve.

The curve y f x completely above x -axis. Adjacent columns will ease copying of the formula. This is the question in my book and I cant seem to get an answer.

If you want to select a definite integral. 821 Integration as the limit of a sum. 824 fxfx 825 Substitution Reverse Chain Rule.

This video explains all about the area under the curve and integration of a curve Thanks for WatchingPlease leave a LIKE to Sup. Now the inter and on RHS for x3 first and then subtract the value of RHS for x3. You can calculate its area easily with this formula.

One application of the definite integral is finding displacement when given a velocity function. Thus the area can be computed using the above formula. Shows a typical rectangle Δx wide and y high.

The area under the curve from x 0 to 1 and the x axis can be found with the equation. The graph made will have a particular upper and lower function within a given range. We will be approximating the amount of area that lies between a function and the x-axis.

Area under polar curve. Start by inserting a helper column in your data set. Area int_ab fx dx The variables above and below the integration symbol a and b are known as the bounds of the integration.

Pin On Integral Calculus

Pin On Math Physics

Find The Area Of The Shaded Region Integral Calculus Example 1 Calculus Region Areas

Derivative Vs Integral Studying Math Learning Math Mathematics Education